Are Ionization Energies Always Negative

7.4: Ionization Free energy

Last updated
Relieve every bit PDF

Page ID: 21740

Learning Objectives

To correlate ionization energies with the chemistry of the elements

We have seen that when elements react, they ofttimes gain or lose enough electrons to achieve the valence electron configuration of the nearest noble gas. Why is this so? In this department, we develop a more than quantitative approach to predicting such reactions past examining periodic trends in the energy changes that back-trail ion germination.

Ionization Energies

Considering atoms do not spontaneously lose electrons, energy is required to remove an electron from an atom to form a cation. Chemists define the ionization energy (\(I\)) of an chemical element as the corporeality of energy needed to remove an electron from the gaseous atom \(Eastward\) in its ground land. \(I\) is therefore the energy required for the reaction

\[ E_{(g)} \rightarrow E^+_{(thou)} +e^- \;\;\ \text{free energy required=I } \characterization{seven.4.i} \]

Because an input of energy is required, the ionization free energy is always positive (\(I > 0\)) for the reaction as written in Equation \(\PageIndex{1}\). Larger values of I mean that the electron is more tightly bound to the cantlet and harder to remove. Typical units for ionization energies are kilojoules/mole (kJ/mol) or electron volts (eV):

\[one\; eV/atom = 96.49\; kJ/mol \nonumber \]

If an atom possesses more than one electron, the amount of energy needed to remove successive electrons increases steadily. We can define a first ionization energy (\(I_1\)), a second ionization free energy (\(I_2\)), and in full general an nth ionization free energy (\(I_n\)) co-ordinate to the post-obit reactions:

\[ \ce{Due east(yard) \rightarrow E^+(grand) +eastward^-} \;\;\ I_1=\text{1st ionization free energy} \characterization{vii.4.2} \]

\[ \ce{E^{+}(m) \rightarrow E^{two+}(m) +east^-} \;\;\ I_2=\text{2nd ionization free energy} \label{seven.4.3} \]

\[ \ce{E^{two+}(g) \rightarrow E^{iii+}(grand) +e^-} \;\;\ I_3=\text{third ionization energy} \characterization{7.4.4} \]

Values for the ionization energies of \(Li\) and \(Be\) listed in Table \(\PageIndex{1}\) show that successive ionization energies for an element increment as they go; that is, information technology takes more energy to remove the 2nd electron from an cantlet than the first, so forth. At that place are ii reasons for this tendency. First, the 2nd electron is beingness removed from a positively charged species rather than a neutral one, so in accordance with Coulomb'due south law, more than energy is required. Second, removing the first electron reduces the repulsive forces among the remaining electrons, so the attraction of the remaining electrons to the nucleus is stronger.

Successive ionization energies for an chemical element increase.

Tabular array \(\PageIndex{ane}\): Ionization Energies (in kJ/mol) for Removing Successive Electrons from Li and Be. Source: Data from CRC Handbook of Chemistry and Physics (2004).
Reaction	Electronic Transition	\(I\)	Reaction	Electronic Transition	\(I\)
\(\ce{Li (k)\rightarrow Li^+ (g) + e^-}\)	\(1s^22s^1 \rightarrow 1s^2\)	I ₁ = 520.2	\(\ce{Be (g) \rightarrow Be^+(g) + eastward^-}\)	\(1s^22s^ii \rightarrow 1s^22s^ane\)	I ₁ = 899.5
\(\ce{Li^+(g) \rightarrow Li^{2+}(one thousand) +e^-}\)	\(1s^2 \rightarrow 1s^1\)	I ₂ = 7298.two	\(\ce{Exist^+(g) \rightarrow Be^{2+}(g) + east^-}\)	\(1s^22s^ane \rightarrow 1s^ii\)	I ₂ = 1757.1
\(\ce{Li^{2+} (g) \rightarrow Li^{3+}(g) + e^-}\)	\(1s^one \rightarrow 1s^0\)	I ₃ = xi,815.0	\(\ce{Be^{2+}(g) \rightarrow Be^{3+}(g) + e^-}\)	\(1s^two \rightarrow 1s^1\)	I ₃ = 14,848.8
			\(\ce{Exist^{three+}(1000) \rightarrow Be^{4+}(g) + eastward^-}\)	\(1s^1 \rightarrow 1s^0\)	I ₄ = 21,006.six

The increase in successive ionization energies, withal, is not linear, but increases drastically when removing electrons in lower \(north\) orbitals closer to the nucleus. The most important consequence of the values listed in Table \(\PageIndex{ane}\) is that the chemistry of \(\ce{Li}\) is dominated past the \(\ce{Li^+}\) ion, while the chemistry of \(\ce{Be}\) is dominated by the +two oxidation state. The free energy required to remove the 2nd electron from \(\ce{Li}\):

\[\ce{Li^+(yard) \rightarrow Li^{ii+}(g) + e^-} \label{7.4.5} \]

is more than 10 times greater than the energy needed to remove the offset electron. Similarly, the energy required to remove the third electron from \(\ce{Be}\):

\[\ce{Be^{two+}(g) \rightarrow Exist^{3+}(g) + e^-} \label{7.4.6} \]

is virtually 15 times greater than the energy needed to remove the starting time electron and around eight times greater than the energy required to remove the second electron. Both \(\ce{Li^+}\) and \(\ce{Be^{2+}}\) have ones ² closed-shell configurations, and much more free energy is required to remove an electron from the 1s ^two core than from the iidue south valence orbital of the aforementioned element. The chemical consequences are enormous: lithium (and all the alkali metals) forms compounds with the i+ ion but non the two+ or three+ ions. Similarly, beryllium (and all the alkaline world metals) forms compounds with the 2+ ion but non the 3+ or iv+ ions. The free energy required to remove electrons from a filled core is prohibitively large and merely cannot exist achieved in normal chemical reactions.

The energy required to remove electrons from a filled core is prohibitively large under normal reaction weather.

Ionization Energy: Ionization Energy, YouTube(opens in new window) [youtu.be] (opens in new window)

Ionization Energies of s- and p-Block Elements

Ionization energies of the elements in the third row of the periodic table exhibit the same blueprint as those of \(Li\) and \(Be\) (Table \(\PageIndex{2}\)): successive ionization energies increase steadily every bit electrons are removed from the valence orbitals (3s or 3p, in this example), followed by an especially large increase in ionization energy when electrons are removed from filled core levels as indicated past the bold diagonal line in Table \(\PageIndex{2}\). Thus in the tertiary row of the periodic tabular array, the largest increase in ionization energy corresponds to removing the fourth electron from \(Al\), the fifth electron from Si, and so forth—that is, removing an electron from an ion that has the valence electron configuration of the preceding element of group 0. This pattern explains why the chemical science of the elements unremarkably involves only valence electrons. Too much free energy is required to either remove or share the inner electrons.

Table \(\PageIndex{2}\): Successive Ionization Energies (in kJ/mol) for the Elements in the Third Row of the Periodic Tabular array.Source: Information from CRC Handbook of Chemical science and Physics (2004).
Element	\(I_1\)	\(I_2\)	\(I_3\)	\(I_4\)	\(I_5\)	\(I_6\)	\(I_7\)
*Inner-crush electron
Na	495.8	4562.4*	—	—	—	—	—
Mg	737.7	1450.vii	7732.seven	—	—	—	—
Al	577.4.4	1816.vii	2744.8	11,577.four.4	—	—	—
Si	786.5	1577.1	3231.6	4355.5	16,090.half dozen	—	—
P	1011.viii	1907.four.4	2914.1	4963.6	6274.0	21,267.4.iii	—
S	999.half dozen	2251.8	3357	4556.2	7004.iii	8495.8	27,107.4.3
Cl	1251.2	2297.vii	3822	5158.vi	6540	9362	11,018.two
Ar	1520.6	2665.9	3931	5771	7238	8781.0	11,995.three

Example \(\PageIndex{1}\): Highest 4th Ionization Energy

From their locations in the periodic table, predict which of these elements has the highest 4th ionization energy: B, C, or North.

Given: 3 elements

Asked for: chemical element with highest fourth ionization energy

Strategy:

List the electron configuration of each element.
Determine whether electrons are being removed from a filled or partially filled valence vanquish. Predict which chemical element has the highest fourth ionization energy, recognizing that the highest energy corresponds to the removal of electrons from a filled electron core.

Solution:

A These elements all prevarication in the second row of the periodic tabular array and have the following electron configurations:

B: [He]2s ²iip ¹
C: [He]2southward ²2p ^two
North: [He]2south ²2p ^three

B The fourth ionization free energy of an chemical element (\(I_4\)) is defined as the energy required to remove the fourth electron:

\[Eastward^{three+}_{(g)} \rightarrow East^{4+}_{(yard)} + due east^- \nonumber \]

Because carbon and nitrogen have four and v valence electrons, respectively, their fourth ionization energies correspond to removing an electron from a partially filled valence beat. The fourth ionization energy for boron, however, corresponds to removing an electron from the filled 1s ² subshell. This should crave much more energy. The actual values are equally follows: B, 25,026 kJ/mol; C, 6223 kJ/mol; and N, 7475 kJ/mol.

Practise \(\PageIndex{1}\): Lowest Second Ionization Energy

From their locations in the periodic tabular array, predict which of these elements has the lowest second ionization free energy: Sr, Rb, or Ar.

Reply: \(\ce{Sr}\)

The kickoff column of data in Table \(\PageIndex{ii}\) shows that commencement ionization energies tend to increment beyond the third row of the periodic table. This is because the valence electrons do not screen each other very well, allowing the effective nuclear charge to increment steadily beyond the row. The valence electrons are therefore attracted more strongly to the nucleus, so diminutive sizes subtract and ionization energies increase. These furnishings represent two sides of the same coin: stronger electrostatic interactions between the electrons and the nucleus further increase the free energy required to remove the electrons.

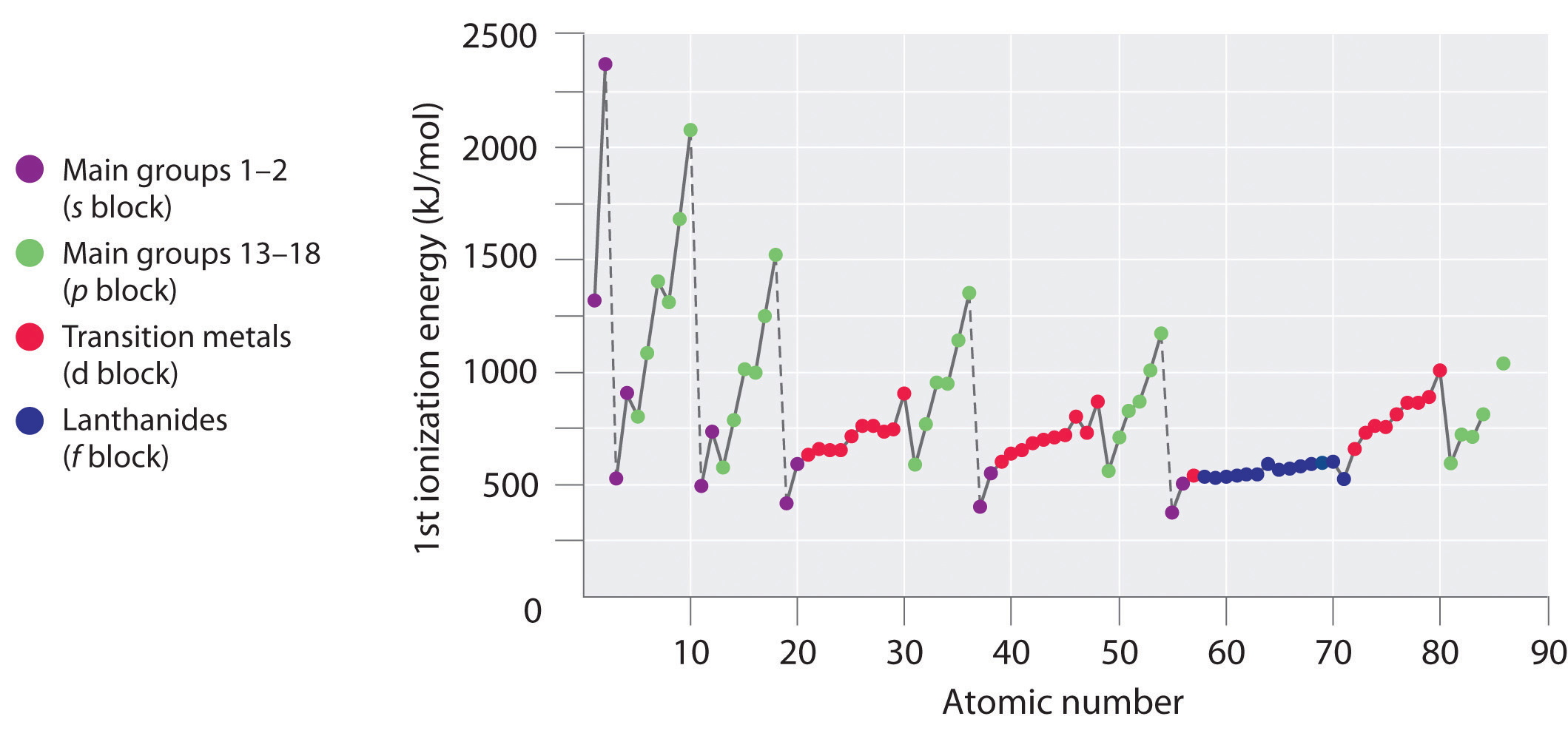
Effigy \(\PageIndex{1}\): A Plot of Periodic Variation of Start Ionization Energy with Diminutive Number for the Outset Six Rows of the Periodic Table. In that location is a decrease in ionization energy inside a group (most easily seen here for groups 1 and 18).

Yet, the first ionization energy decreases at Al ([Ne]3s ²3p ^one) and at Due south ([Ne]iiis ⁱⁱ3p ⁴). The electron configurations of these "exceptions" provide the answer why. The electrons in aluminum's filled 3southward ² subshell are better at screening the 3p ¹ electron than they are at screening each other from the nuclear charge, so the south electrons penetrate closer to the nucleus than the p electron does and the p electron is more easily removed. The decrease at S occurs because the two electrons in the same p orbital repel each other. This makes the S atom slightly less stable than would otherwise be expected, as is true of all the grouping 16 elements.

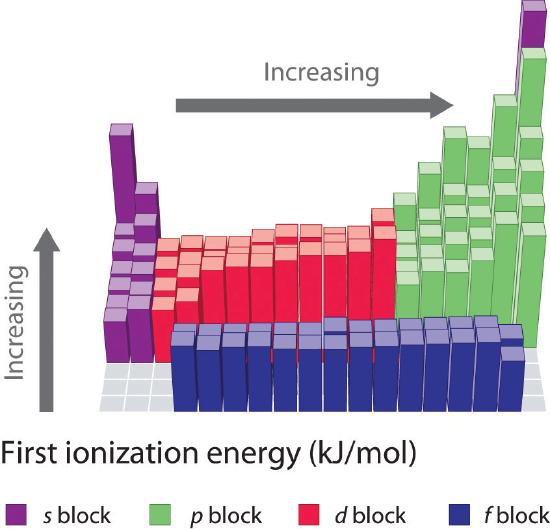
Figure \(\PageIndex{ii}\): First Ionization Energies of the *due south*-, p-, d-, and f-Block Elements

The first ionization energies of the elements in the first half-dozen rows of the periodic table are plotted in Figure \(\PageIndex{1}\) and are presented numerically and graphically in Figure \(\PageIndex{2}\). These figures illustrate three important trends:

The changes seen in the second (Li to Ne), 4th (K to Kr), fifth (Rb to Xe), and 6th (Cs to Rn) rows of the s and p blocks follow a design similar to the pattern described for the third row of the periodic table. The transition metals are included in the fourth, fifth, and sixth rows, however, and the lanthanides are included in the sixth row. The first ionization energies of the transition metals are somewhat similar to i another, as are those of the lanthanides. Ionization energies increase from left to right across each row, with discrepancies occurring at ns ² np ^one (group xiii), ns ² np ^four (group 16), and ns ⁱⁱ(north − 1)d ^x (grouping 12).
First ionization energies generally decrease down a column. Although the principal quantum number n increases downwardly a column, filled inner shells are constructive at screening the valence electrons, then there is a relatively minor increase in the effective nuclear charge. Consequently, the atoms become larger equally they larn electrons. Valence electrons that are farther from the nucleus are less tightly bound, making them easier to remove, which causes ionization energies to decrease. A larger radius typically corresponds to a lower ionization energy.
Because of the first ii trends, the elements that form positive ions most hands (have the lowest ionization energies) prevarication in the lower left corner of the periodic table, whereas those that are hardest to ionize lie in the upper right corner of the periodic table. Consequently, ionization energies generally increase diagonally from lower left (Cs) to upper correct (He).

The darkness of the shading inside the cells of the tabular array indicates the relative magnitudes of the ionization energies. Elements in gray have undetermined outset ionization energies. Source: Data from *CRC Handbook of Chemistry and Physics* (2004).

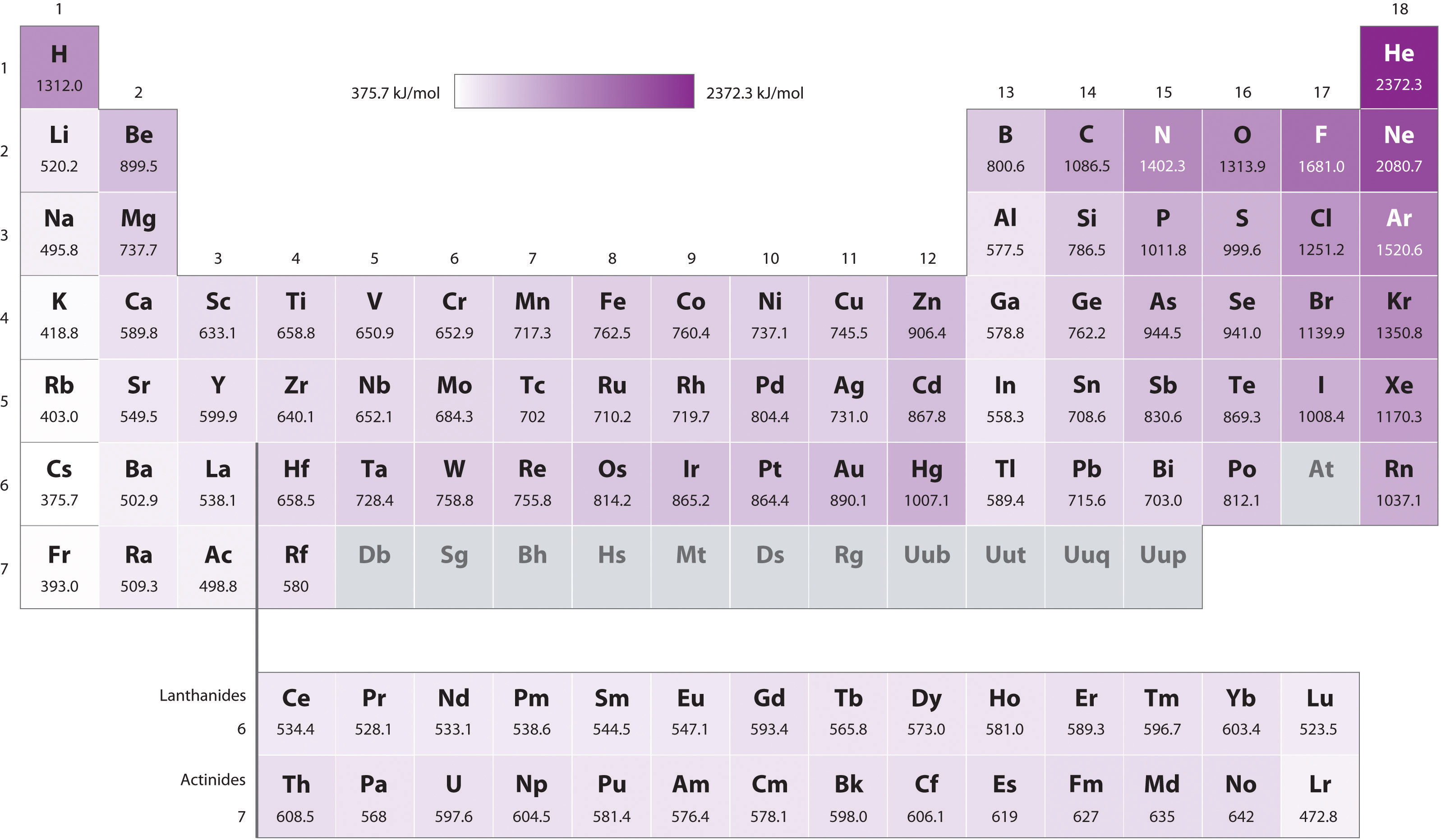
Generally, \(I_1\) increases diagonally from the lower left of the periodic table to the upper right.

Gallium (Ga), which is the outset element following the first row of transition metals, has the following electron configuration: [Ar]fours ²3d ¹⁰4p ¹. Its kickoff ionization energy is significantly lower than that of the immediately preceding element, zinc, because the filled 3d ¹⁰ subshell of gallium lies inside the fourp subshell, shielding the unmarried 4p electron from the nucleus. Experiments have revealed something of even greater involvement: the second and tertiary electrons that are removed when gallium is ionized come up from the fours ² orbital, non the 3d ^x subshell. The chemistry of gallium is dominated by the resulting Ga^three ⁺ ion, with its [Ar]3d ^x electron configuration. This and like electron configurations are peculiarly stable and are often encountered in the heavier p-block elements. They are sometimes referred to as pseudo element of group 0 configurations. In fact, for elements that exhibit these configurations, no chemic compounds are known in which electrons are removed from the (northward − one) d ¹⁰ filled subshell.

Ionization Energies of Transition Metals & Lanthanides

Every bit nosotros noted, the first ionization energies of the transition metals and the lanthanides change very fiddling across each row. Differences in their 2nd and third ionization energies are as well rather small, in sharp dissimilarity to the blueprint seen with the s- and p-block elements. The reason for these similarities is that the transition metals and the lanthanides grade cations past losing the ns electrons before the (north − 1)d or (north − 2)f electrons, respectively. This means that transition metal cations take (n − 1)d ⁿ valence electron configurations, and lanthanide cations have (n − 2)f ⁿ valence electron configurations. Because the (n − 1)d and (northward − 2)f shells are closer to the nucleus than the ns shell, the (n − 1)d and (north − 2)f electrons screen the ns electrons quite effectively, reducing the effective nuclear charge felt by the ns electrons. As Z increases, the increasing positive charge is largely canceled past the electrons added to the (n − 1)d or (n − 2)f orbitals.

That the ns electrons are removed earlier the (due north − ane)d or (n − two)f electrons may surprise yous considering the orbitals were filled in the reverse order. In fact, the ns, the (n − i)d, and the (n − ii)f orbitals are so close to one another in energy, and interpenetrate ane some other then extensively, that very small-scale changes in the effective nuclear accuse can modify the social club of their energy levels. As the d orbitals are filled, the constructive nuclear charge causes the threed orbitals to be slightly lower in energy than the ivs orbitals. The [Ar]threed ² electron configuration of Ti² ⁺ tells us that the fourdue south electrons of titanium are lost before the iiid electrons; this is confirmed by experiment. A similar pattern is seen with the lanthanides, producing cations with an (n − 2)f ^{due north} valence electron configuration.

Because their first, second, and third ionization energies change so little across a row, these elements take important horizontal similarities in chemical properties in addition to the expected vertical similarities. For instance, all the get-go-row transition metals except scandium form stable compounds every bit M²⁺ ions, whereas the lanthanides primarily form compounds in which they exist as M^three+ ions.

Case \(\PageIndex{two}\): Lowest Outset Ionization Energy

Use their locations in the periodic table to predict which element has the lowest first ionization energy: Ca, Chiliad, Mg, Na, Rb, or Sr.

Given: six elements

Asked for: element with lowest first ionization energy

Strategy:

Locate the elements in the periodic table. Based on trends in ionization energies across a row and downwards a column, identify the element with the lowest starting time ionization energy.

Solution:

These six elements grade a rectangle in the two far-left columns of the periodic table. Because we know that ionization energies increment from left to correct in a row and from bottom to tiptop of a column, we tin can predict that the chemical element at the bottom left of the rectangle will take the lowest first ionization free energy: Rb.

Exercise \(\PageIndex{2}\): Highest First Ionization Energy

Use their locations in the periodic table to predict which chemical element has the highest showtime ionization energy: Equally, Bi, Ge, Pb, Sb, or Sn.

Answer: \(\ce{Every bit}\)

Summary

The tendency of an chemical element to lose electrons is one of the most of import factors in determining the kind of compounds it forms. Periodic behavior is about evident for ionization free energy ( I ), the energy required to remove an electron from a gaseous atom. The energy required to remove successive electrons from an atom increases steadily, with a substantial increase occurring with the removal of an electron from a filled inner shell. Consequently, merely valence electrons can be removed in chemical reactions, leaving the filled inner shell intact. Ionization energies explain the common oxidation states observed for the elements. Ionization energies increment diagonally from the lower left of the periodic tabular array to the upper right. Minor deviations from this trend can exist explained in terms of peculiarly stable electronic configurations, called pseudo noble gas configurations, in either the parent cantlet or the resulting ion.